Number Sets
Number Sets: Learn
For many aspect of mathematics and algebra, it is useful to be able to discuss a subset of all the known numbers. Here are the most common sets.
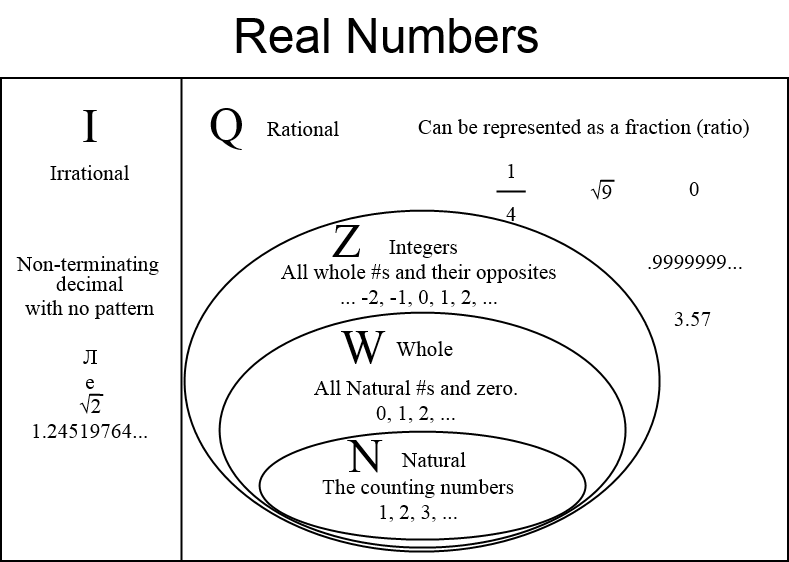
- Natural Numbers are the normal whole numbers used for counting and ordering, starting with 1, 2, 3, ... (Think: what's the most natural thing we do with numbers? The very first thing you learn to do with them? Count!)
-
An Ordinal Number is a natural number used for ordering
(1st, 2nd, 3rd, ...). -
A Whole Number is any of the counting numbers, as well as zero. You
can make a list of Whole Numbers starting at zero
(0, 1, 2, ...)
This means all natural numbers are also whole numbers. However, zero is the only number that is a whole number but NOT a natural number. -
The Integers are the positive and negative whole numbers
(..., -3, -2, -1, 0, 1, 2, 3, ...)
This means that all whole numbers are also integers. But the negative integers are NOT whole numbers. Sometimes you might also see the whole numbers described as "non-negative integers" - Rational Numbers are the numbers which can be represented by a fraction (it comes from the word "ratio" and ratios can be written in fraction form!). That means that all fractions are rational numbers, and also all integers are rational, too (they can be written as a fraction with denominator of 1).
- Irrational Numbers are the numbers which cannot be represented by a fraction. You might recognize them by their decimals, which never end and have no repeating pattern. The number PI is irrational, and so are square roots of non-square numbers (that is, square root of 2 is irrational, but square root of 9 evaluates to 3, which is rational).
- The Real Numbers include all of the rational and irrational numbers. All real numbers are either rational or irrational.
The following diagram may help you organize and identify numbers into
their proper sets.
Number Sets: Practice
Choose the most specific number set for the given number or definition.
00:00
Press the Start Button To Begin
You have
0
correct and
0
incorrect.
This is
0
percent correct.
Play
| Game Name | Description | Best Score |
|---|---|---|
| How many correct answers can you get in 60 seconds? | 0 | |
|
Extra time is awarded for each correct answer.
Play longer by getting more correct. |
0 | |
| How fast can you get 20 more correct answers than wrong answers? | 999 |
Explore
Math Lessons by Grade
Math Topics
- Addition
- Algebra
- Comparing
- Counting
- Decimals
- Division
- Equations
- Estimation and Mental Math
- Exponents
- Fractions
- Geometry
- Measurement
- Money
- Multiplication
- Naming Numbers
- Patterns
- Percents and Ratios
- Place Value
- Properties
- Statistics
- Subtraction